GMAT Explanation, Video Solution, and Additional Practice!
For a certain race, 3 teams were allowed to enter 3 members each. A team earned 6 – n points whenever one of its members finished in nth place, where 1 ≤ n ≤ 5. There were no ties, disqualifications, or withdrawals. If no team earned more than 6 points, what is the least possible score a team could have earned?
(A) 0
(B) 1
(C) 2
(D) 3
(E) 4
Here’s another one from the GMAT Prep Software free tests 1 and 2 so better to wait on this explanation unless you’ve done those tests already. This is another GMAT puzzle that relies on you being organized. You basically need to make a list of the possibilities keeping track of the constraints with the goal of maximizing the points for two of the teams so the third team has the least possible score. In terms of the zoomed out approach this question reminds of this is other GMAT puzzle: The number 75 can be written as the sum of the squares of 3 different positive integers
Here’s the quick solution and we’ll go in depth below:
Define the question
Table of Contents
What is the least possible score a team could have earned? Ok, so we’re aiming for the least for one of the teams. So what does that mean for the other teams? Maximize them!
Setup
Let’s review our constraints:
-3 teams were allowed to enter 3 members each.
-A team earned 6 – n points whenever one of its members finished in nth place
-1 ≤ n ≤ 5.
-No team earned more than 6 points
-There were no ties, disqualifications, or withdrawals.
So, follow the above rules while trying to maximize 2 of the teams (try for 6 points each) to make the least possible score for the third team.
Solve
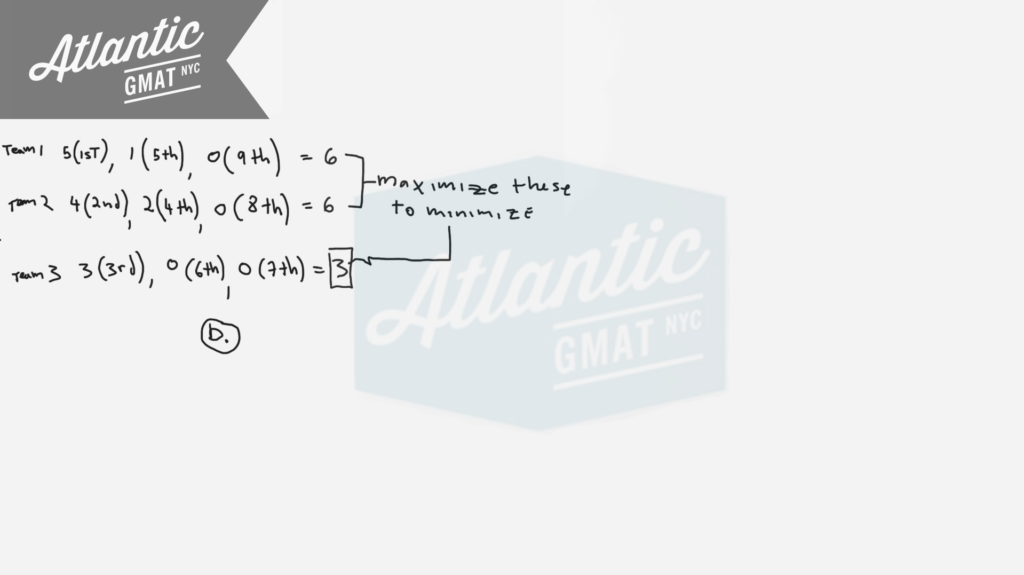
Team 1
1st place = 5 points
then Team 1 can only earn 1 more point (no team earned more than 6 points)
5th place = 1 point
That’s the max 6 so the last member team 1 needs to finish in a place that earns no points
9th place = 0 points
Team 1 total points = 6
Team 2
2nd place = 4 points
then Team 2 can only earn 2 more points
4th place = 2 points
That’s the max 6 so the last member of team 2 needs to finish in a place that earns no points
8th place = 0 points
Team 2 total points = 6
Team 3 has everything that’s left
3rd place = 3 points
5th place = 0 points
6th place = 0 points
Team 3 total points = 3
Video Solution: For a certain race 3 teams were allowed…
Additional GMAT Max/Min Puzzle Example Questions
Two questions from to mind. They’re both different from this one and different from each other but share this crucial max/min idea:
A certain city with population of 132,000 is to be divided into 11 voting districts
Seven pieces of rope have an average
And here is another max/min example from GMAT question of the day.