For which of the following functions is f(a+b) = f(b) + f(a) for all positive numbers a and b?

A. f(x) =
B. f(x) = x+1
C. f(x) = √x
D. f(x) = 2/x
E. f(x) = -3x

Deja vu! Here’s another function question from the GMAT prep tests 1 and 2 (extremely similar to this one: For which of the following functions f(x) = f(1-x)). Also, for a more in depth discussion of the topic here’s our main page for GMAT Functions.

Below we’ll define the question and then lay out an explanation. At the bottom of the page you will also find a hand written explanation that resembles more what I would do on test day and an explanation video. Then, finally, links to additional functions practice. All bases covered. You will get this!

Functions are easier than they look (take your time defining the question)

The first read is usually confusing. Just keep in mind that the premise is simple. Take your time to let all of the information sink in so you can define what you’re looking for in basic terms.

For this one we need to: find the answer choice for which plugging in a + b into the function is the same as first plugging in a then plugging in b and summing the outcome.

Solve by picking numbers and plugging into the answer choices (often how you will solve these functions)

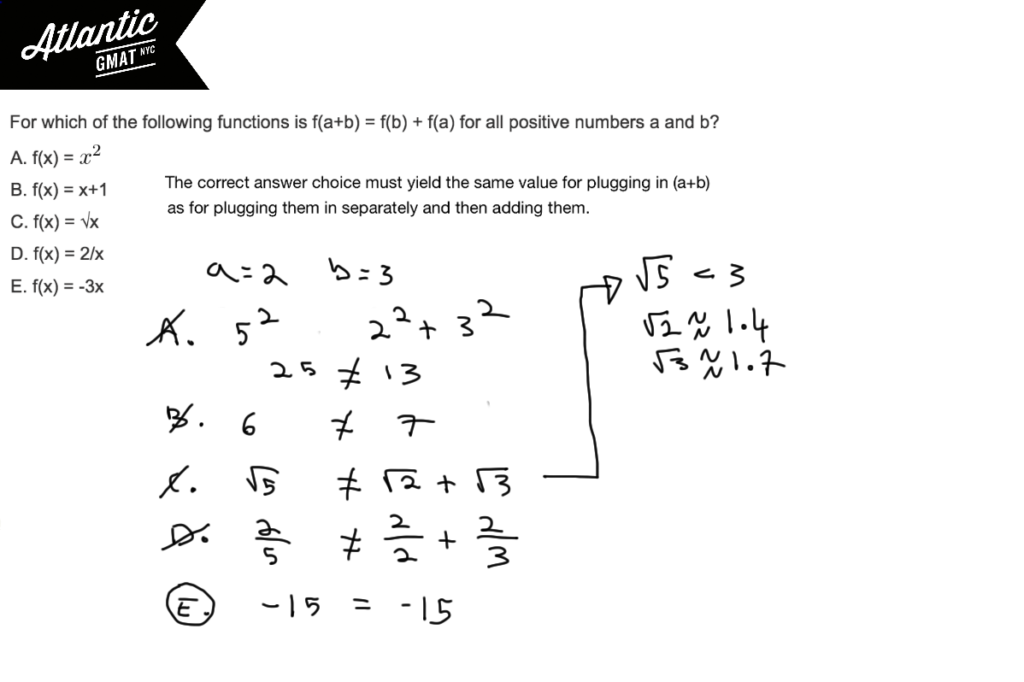
Let’s just go through the answer choices. I would pick values for a and b. Let’s go for a = 2, b = 3. Just a recap we want f(2 + 3) = f(2) + f(3).

A. f(x) =

vs

25 vs 13

B. f(x) = x+1

5 + 1 vs (2+1) + (3+1)

6 vs 7

C. f(x) = √x

√5 vs. √2 + √3

√5 is less than 3. √2 is about 1.4, √4 is about 1.7 so their sum is more than 3.

D. f(x) = 2/x

2/5 vs 2/2 + 2/3

The right side is clearly bigger.

E. f(x) = -3x

-3(5) vs -3(2) + -3(3)

-15 = -15

Here is the above condensed into a diagram that more closely resembles how I would approach it on a test

Video solution: For which of the following functions is f(a+b) = f(b) + f(a) for all positive numbers a and b?

Additional GMAT Functions Practice Questions!

Here’s an extremely challenging GMAT function question that’s a bit different and more on the puzzle side of things. I skipped it the first time around but found a relatively easy way to solve it using a basic but extremely important divisibility rule that every person studying for the GMAT should know: For every positive even integer n, the function h(n) is defined to be the product of all the even integers from 2 to n, inclusive. If p is the smallest prime factor of h(100) +1, then p is?

This question is nearly identical to the one above so great practice: For which of the following functions f is f(x) = f(1-x) for all x?

And one more similar-ish challenging function question from the GMAT Prep Tests: If the operation @ is defined for all integers a and b by a@b = a+b – ab, which of the following statements must be true for all integers a, b and c?