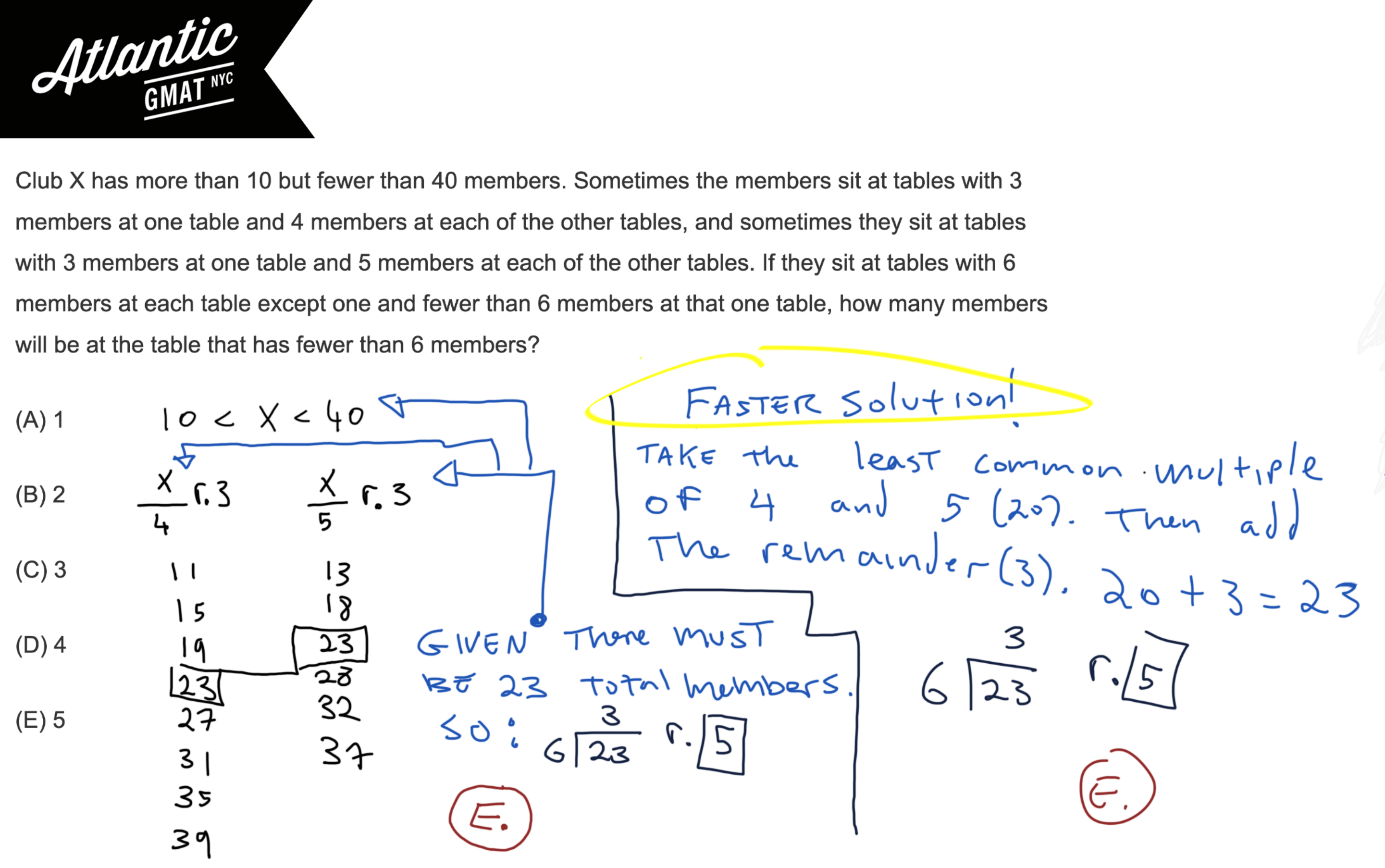
Club X has more than 10 but fewer than 40 members. Sometimes the members sit at tables with 3 members at one table and 4 members at each of the other tables, and sometimes they sit at tables with 3 members at one table and 5 members at each of the other tables. If they sit at tables with 6 members at each table except one and fewer than 6 members at that one table, how many members will be at the table that has fewer than 6 members? GMAT Explanation
Club X has more than 10 but fewer than 40 members. Sometimes the members sit at tables with 3 members at one table and 4 members at each of the other tables, and sometimes they sit at tables with 3 members at one table and 5 members at each of the other tables. If they sit at tables with 6 members at each table except one and fewer than 6 members at that one table, how many members will be at the table that has fewer than 6 members?
(A) 1
(B) 2
(C) 3
(D) 4
(E) 5
This is a tough question from the GMAT Official Guide that we almost always use as an example in our divisibility/remainder lesson. Without any setup most GMAT tutoring students get lost. They start out trying to do some sort of algebra and then fizzle out. Good news: there’s a very easy way to tackle these (even though this style of question is considered difficult).
So let’s start by reading carefully and defining the question: How many members will be at the table that has fewer than 6 members? Translate: what’s the remainder when the total number of people is divided by 6?
So somehow we need to figure out the total number of members in Club X. What else do we have:
Sometimes the members sit at tables with 3 members at one table and 4 members at each of the other tables: When the total is divided by 4 the remainder is three.
Sometimes they sit at tables with 3 members at one table and 5 members at each of the other tables: When the total is divided by 5 the remainder is also three.
So now we have enough information to pinpoint the total. We know that it’s a number between 10 and 40 which when divided by 4 or 5 has reminder 3. One practical way to approach this is to make a list of numbers that are remainder 3 when divided by 4 and another list that is remainder 3 when divided by 5. Then see at what number between 10 and 40 is in both lists.
Remainder 3/Divided by 4: 3, 7, 11, 15, 19, 23, 27, 31, 35, 39
Remainder 3/Divided by 5: 3, 8, 13, 18, 23, 28, 33, 38
The overlap is 23. So there must be a total of 23 members in Club X. 23 divided by 6 has a remainder 5.
How many members will be at the table that has fewer than 6 members? There will be 5 members at the table that has fewer than 6 members.
Making a list and cross-referencing is a great way to do these divisibility/remainder questions with members divided amongst tables, children assigned to classrooms, marching bands divided into rows… There is a much faster way though.
Take the least common multiple of the two numbers that you’re dividing by, in this case 4 and 5, and then add the remainder, 3. So the LCM of 4 and 5 is 20. Add 3. 23. For numbers that don’t share factors the LCM is just their product. Easy right?
These questions with people/things being divided into different equal numbered subgroups with some number left over often look challenging because there’s usually a big block of text but, provided that you understand the above, are actually pretty simple and can be answered very quickly with very little calculation. These are great to get on an exam.
Video Solution: Club X has more than 10 but fewer than 40 members
Additional Divisibility/Remainder GMAT Practice Questions
You see these done both ways, with a story or more broken down. This example is spot-on content-wise but a more broken down example: GMAT Question of the Day Divisibility/LCM/Reminder Example
Here’s another word problem divisibility question from Question of the Day for which you need to do some step by step practical work