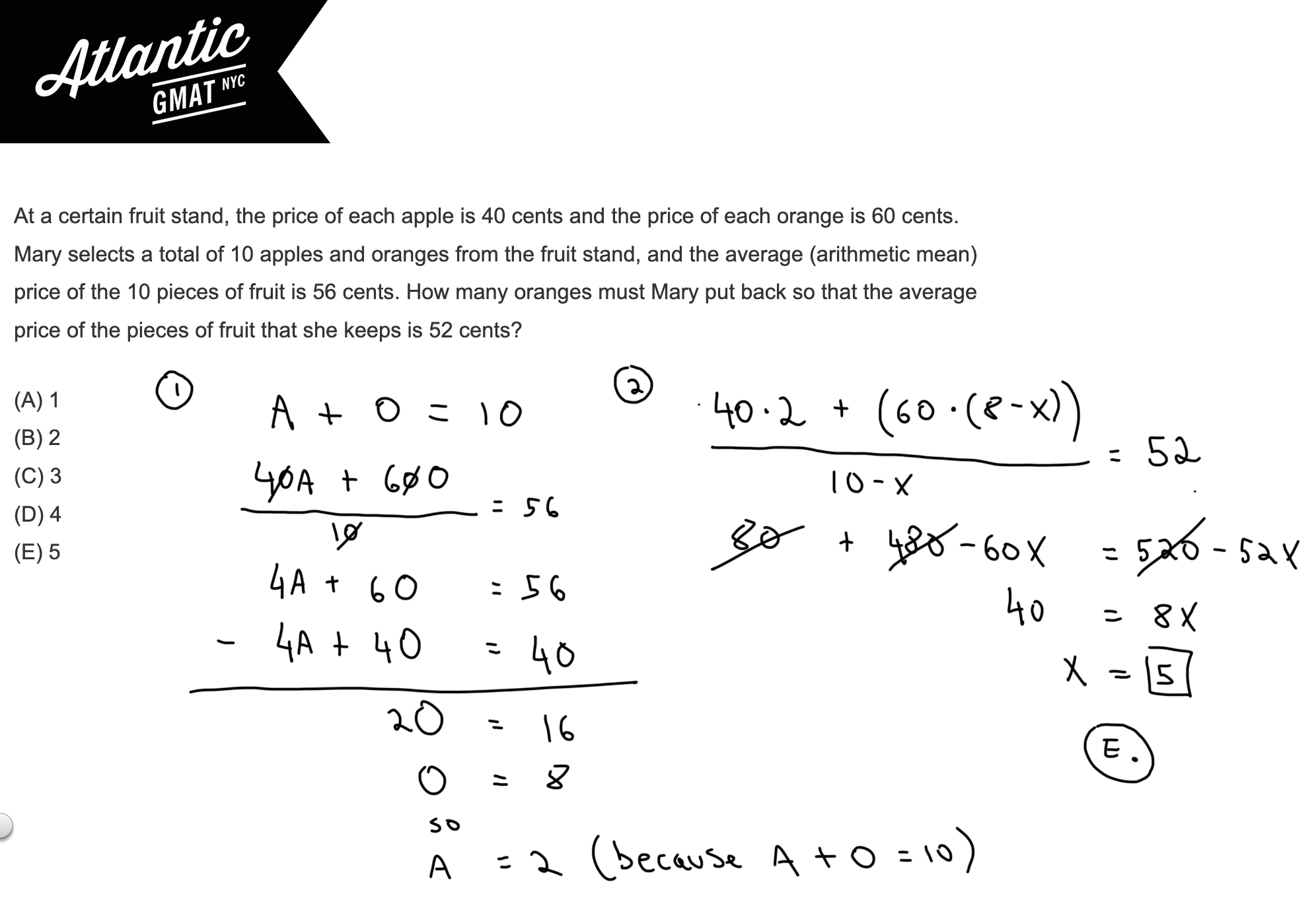
At a certain fruit stand, the price of each apple is 40 cents and the price of each orange is 60 cents. Mary selects a total of 10 apples and oranges from the fruit stand, and the average (arithmetic mean) price of the 10 pieces of fruit is 56 cents. How many oranges must Mary put back so that the average price of the pieces of fruit that she keeps is 52 cents? GMAT Explanation
At a certain fruit stand, the price of each apple is 40 cents and the price of each orange is 60 cents. Mary selects a total of 10 apples and oranges from the fruit stand, and the average (arithmetic mean) price of the 10 pieces of fruit is 56 cents. How many oranges must Mary put back so that the average price of the pieces of fruit that she keeps is 52 cents?
(A) 1
(B) 2
(C) 3
(D) 4
(E) 5
Here’s another word problem from the GMAT official guide (Video solution below). It’s a basic weighted average/system of equations but often people get tripped up defining the equations or stall mid-way because it all feels too complicated. Let’s just take it one step at a time!
First step: read carefully. Don’t rush. Don’t skim. Don’t trail off at the end of sentences. If something doesn’t make sense re-read it. If you think about it for a little and it still doesn’t click you can keep reading to see if more context helps to shed some light. If the lightbulb still isn’t going off that’s OK, once you start getting things organized it’s possible that the puzzle pieces will fall into place.
So now define the question: How many oranges must Mary put back so that the average price of the pieces of fruit that she keeps is 52 cents?
We’re not solving for the number of oranges but the number that Mary must put back in order to decrease the average price to 52 cents. So we’re changing the number of oranges to manipulate the price. OK, so now let’s get our system of equations defined. Start with whatever is easiest for you.
Mary selects a total of 10 apples and oranges from the fruit stand (this looks easiest to me)
A + O = 10
The average (arithmetic mean) price of the 10 pieces of fruit is 56 cents
(40A + 60O)/10 = 56 We can simplify the fraction. 4A + 6O = 56
Now you have a basic system of equations. You can solve via substitution or by adding/subtracting equations. Usually adding/subtracting is easier/faster than substitution. We’re going to subtract:
4A + 6O = 56
4A + 4O = 40
2O = 16
O = 8
We have 8 oranges and 2 apples. So we need to set up an equation for a weighted average that equals 52. We’re going to define the oranges Mary must put back as X. That has to be subtracted from the oranges in the numerator AND the total in denominator. That’s a major pain point in this question, forgetting the remove to oranges from the total on the bottom.
(2*40 + (8 – X)60)/(10 – X) = 52
Now we solve for X.
80 + 480 – 60X = 520 – 52X
40 = 8X
X = 5
For the average price of the pieces of fruit that she keeps to be 52 cents Mary must put 5 oranges back.
We can also error check that. (2*40 + 3*60)5 = 260/5 = 52
I solved it slightly differently in the video. I prefer the solution here but the video is still a good reference.
Additional Weighted Average Practice
Here’s another weighted average question from GMAT Question of the Day