Set S consists of five consecutive integers, and set T consists of seven consecutive integers. Is the median of the numbers in set S equal to the median of the numbers in set T? GMAT Explanation +  Additional Examples!

Set S consists of five consecutive integers, and set T consists of seven consecutive integers. Is the median of the numbers in set S equal to the median of the numbers in set T?

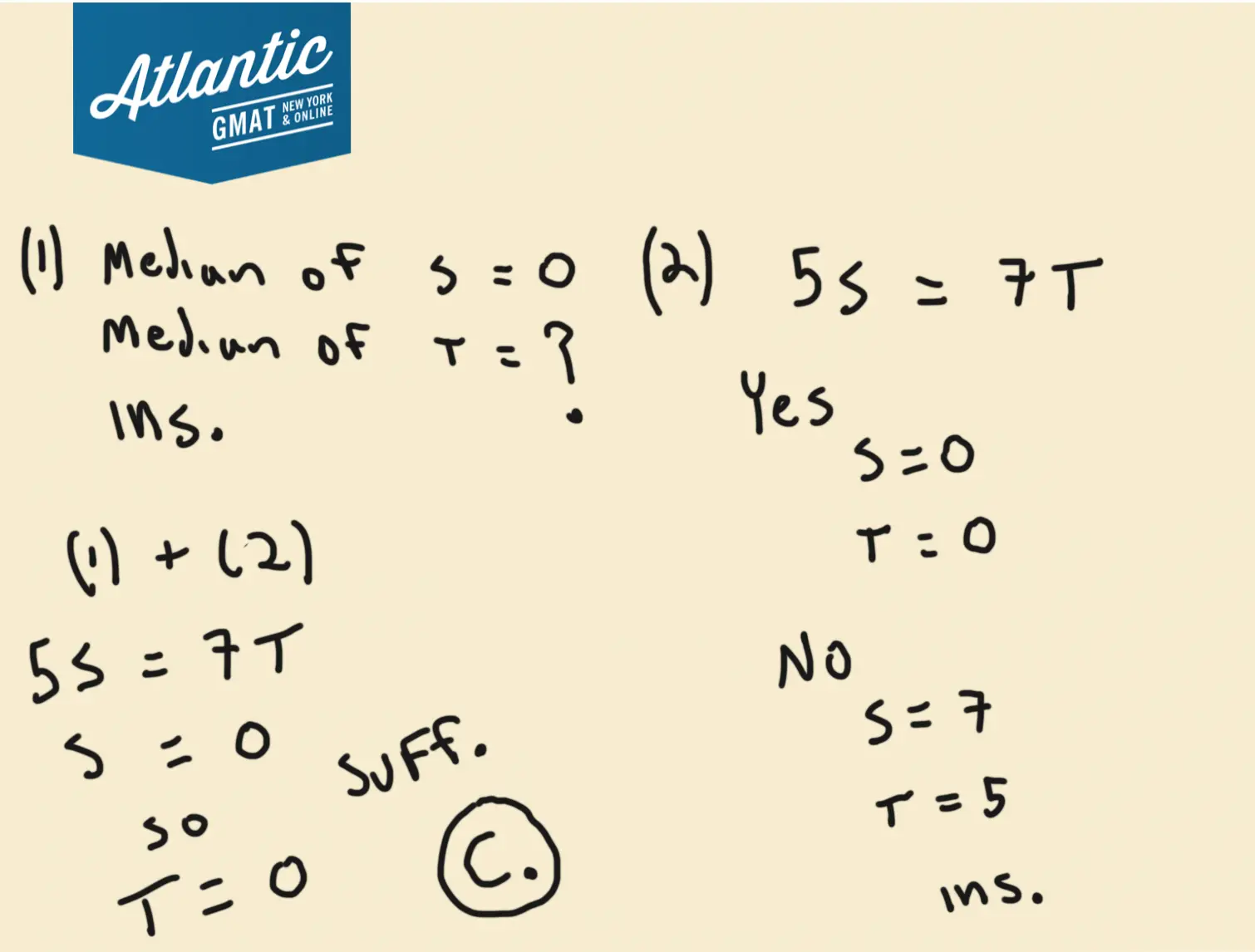
(1) The median of the numbers in Set S is 0
(2) The sum of the numbers in set S is equal to the sum of the numbers in set T

Define the question: Is the median of the numbers in set S equal to the median of the numbers in set T? 

Not a lot to define here, but starting with the question is a great habit to get into. In this case let’s keep median (add link defining median) in mind and remember that it’s a spatial measurement (the middle number of a set).

Statement 1: The median of the numbers in Set S is 0 

What does this tell us about set T? 

Right: absolutely nothing. 

The median of set T could be anything so it’s not possible to determine whether the median of the numbers in set S is equal to the median of the numbers in set T.

INSUFFICIENT. 

Cross out options A and D. 

Statement 2: The sum of the numbers in set S is equal to the sum of the numbers in set T

Remember, in data sufficiency questions, we’re aiming to prove the statements insufficient. 

So, think in terms of Yes/No. Try to set up a scenario that answers “Yes” to the question and a scenario that answers “No”.

In this case let’s follow the rule outlined in statement two while trying to get the medians equal (Yes) and then not equal (No).

Let’s say

Median of set S = s

Median of set T = t

Another way to write that the sum of the sets is the same is: 5s = 7t

Why? Because in an evenly spaced set the sum of the set is equal to the product of the median (or mean) and the number of numbers in the set.

For example:

1, 2, 3

2 is the median. There are three numbers in the set. 

2*3 = 6. 

1 + 2 + 3 = 6.

Also, in an evenly spaced set: the median = the mean. For GMAT quant it’s important to know this rule.

Yes/No

In order to follow statement 2 we have to satisfy this equation: 5s = 7t. So pick numbers for s and t that balance the equation. Let’s start with 7 for “s” and 5 for “t” because 5×7 = 7×5!

No 

s = 7

t = 5

In this case the medians (7 and 5) are different.

Let’s remember with picking values that it’s always worth thinking about some special numbers: 0, 1, -, +, fractions.

0 helps quite a bit because if you make the s and t equal 0 then the medians are the same and the equation is balanced.

Yes

s = 0

t = 0

We have a Yes and a No so statement 2 is insufficient.

Working together?

From statement 1, we know that “s” is equal to 0.

From statement 2, we know that 5s = 7t.

In our statement 2 solutions we already showed that when s = 0 so is t. So that’s a “Yes”. The medians are equal.

Here’s the algebra worked out:

5(0) = 7t

0 = 7t

t = 0

Statements 1 and 2 together are SUFFICIENT. 

Correct answer: C

Here’s the entire solution in a more compact format