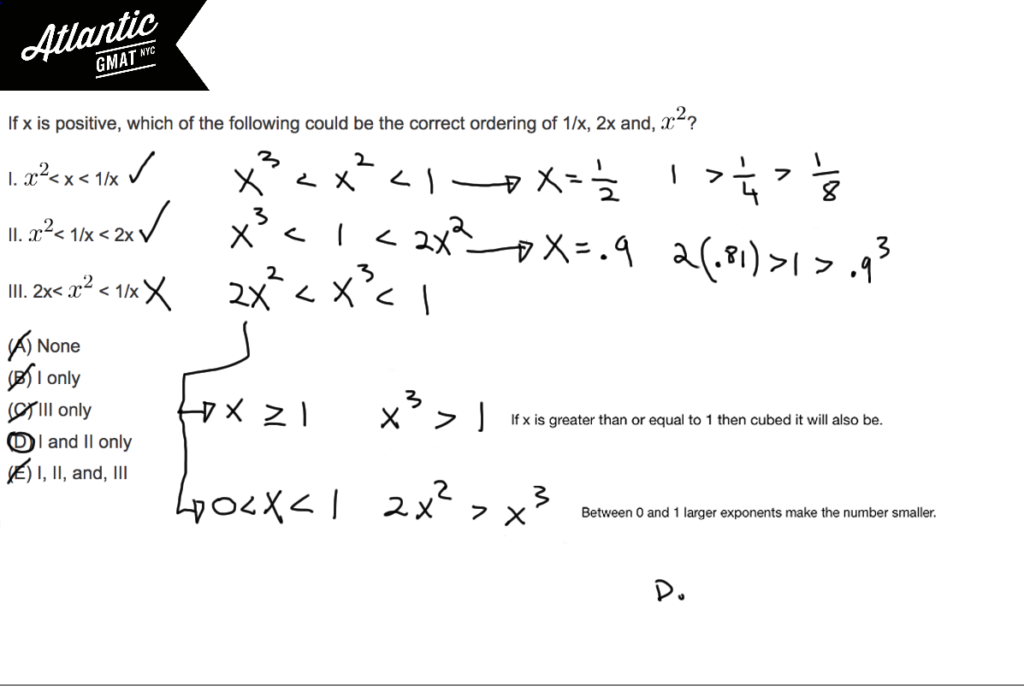
If x is positive, which of the following could be the correct ordering of 1/x, 2x, and, ?
I. < x < 1/x
II. < 1/x < 2x
III. 2x< < 1/x
(A) None
(B) I only
(C) III only
(D) I and II only
(E) I, II, and, III
GMAT roman numeral questions have a bad rap. The GMAT rumor mill has it that these questions are challenging. Some of them might be. Any question type, question structure, or content area can be presented in a difficult way. But I wouldn’t paint roman numeral questions with the broad brush of toughness as I would something very specific for instance: roman numeral, inequality, multi-variable. These three qualities together usually = challenge. As do data sufficiency, long word problem, inequality, multi-variable. You get the picture. There are certain combinations that tend to be challenging, time consuming or both. But there’s generally not a broad category that’s in itself challenging. Back to the task at hand. In order to determine the correct ordering I would be thinking about number picking. I’d focus on big integers, small ones, and numbers between 0 and 1 (we don’t have to worry about negative numbers because x is positive). You may not need to use every one of these options for every choice. I’m just presenting the types of numbers that tend to create different outcomes. You can find a video explanation here: If x is positive, which of the following could be the correct ordering of 1/x, 2x and, x^2?
Note that I did some algebra. Why? We are given x > 0 so that makes it possible to multiple x through to get rid of the fraction in each on of the inequalities. It’s usually easier to deal with “flat” inequalities so if you can, get rid of the fractions. That said, if you don’t know the sign of x you can’t multiply or divide because you won’t know which way to point the inequality. By picking any number between 0 and 1 roman numeral one will work as x will be less than 1 and will be less than
because the larger exponent produces the smaller number (think 1/2*1/2*1/2 vs. 1/2*1/2). Statement two works with numbers between 0 and 1 but at the top end of the scale. They have to big enough so that when squared they are still bigger than 1/2. Statement three doesn’t work. Ever. For positive integers
is always equal to or greater than 1. For numbers between 0 and 1
will always be bigger than
so
will also be bigger than
. Comment away with questions or concerns!