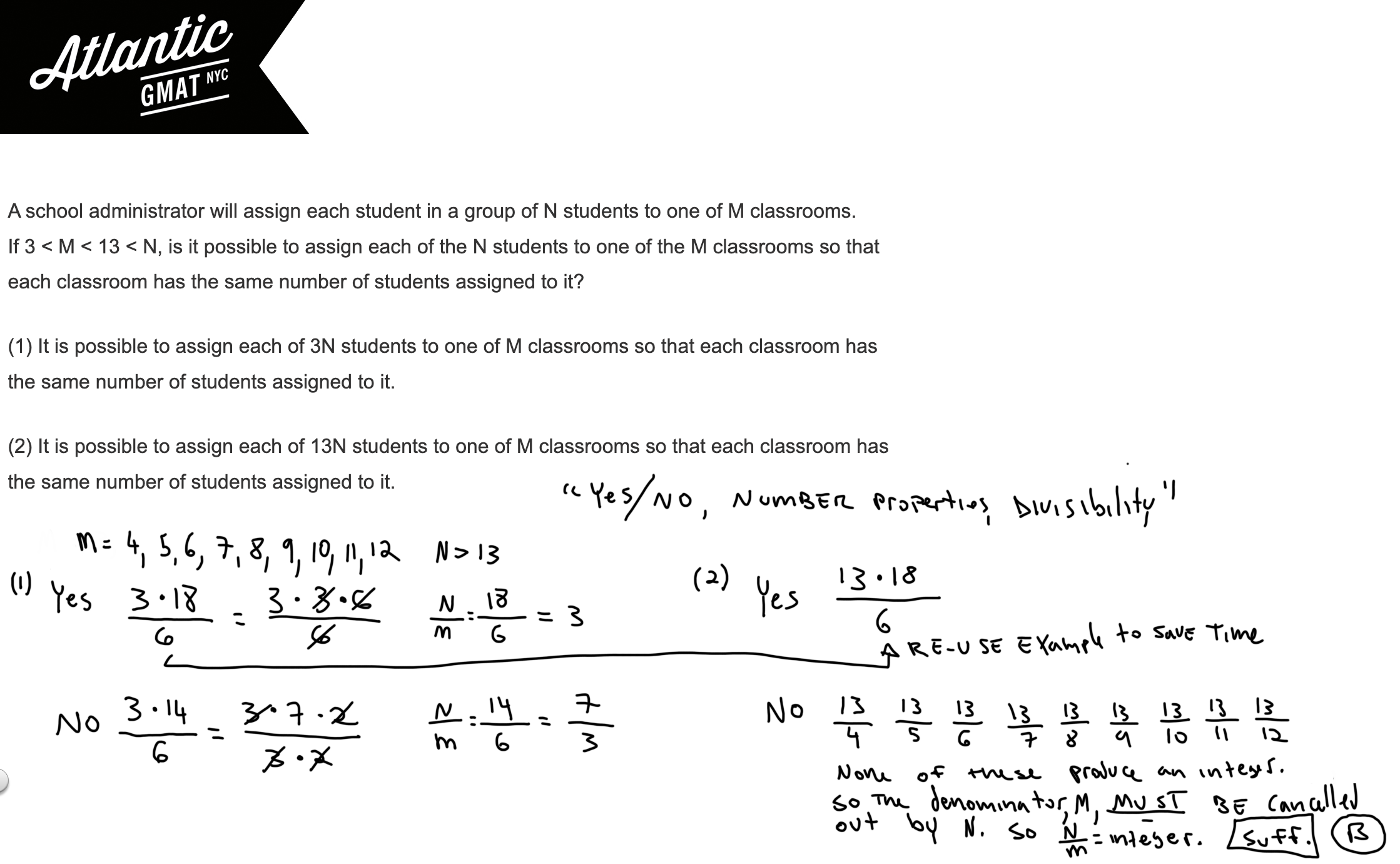
(1) It is possible to assign each of 3N students to one of M classrooms so that each classroom has the same number of students assigned to it.
(2) It is possible to assign each of 13N students to one of M classrooms so that each classroom has the same number of students assigned to it.
A school administrator GMAT Explanation
Table of Contents
This is a super tricky GMAT number properties/divisibility question from the GMAT official guide. Oddly we don’t see this one a lot in GMAT tutoring sessions but maybe we can credit the video explanation for that.
As always let’s start by reading carefully and figuring out the question: is it possible to assign each of the N students to one of the M classrooms so that each classroom has the same number of students assigned to it?
OK, we know that if it has a question mark at the end it’s a question BUT what does that actually mean? Especially on Data Sufficiency you really need to do a step further. In this case the question is, does M divide evenly into N. Or, is N divisible by M. Or is N/M an integer. However way you want to look at it.
The point is: dig into Data Sufficiency information and try to make an inference, organize, or transform in some way. Dig!!!
OK, so now we’ve got the question, and if we had the values for M and N it would be very simple. Of course, we don’t have the values but in the statements we’ll be given constraints which will potentially narrow things down to sufficiency. We do have a constraint in the questions stem though: M is between 3 and 13 and N is greater than 13.
For M that’s quite a narrow range so I might just write values out: 4, 5, 6, 7, 8, 9, 10, 11, 12. Boom! Easy organization.
Let’s get to the statements:
(1) It is possible to assign each of 3N students to one of M classrooms so that each classroom has the same number of students assigned to it.
What does that mean??? 3N/M is an integer. How does that relate to N/M. Let’s try to do a Yes/NO.
M = 4, 5, 6, 7, 8, 9, 10, 11, 12 N > 13
Yes
Pick a value for M and then pick something for N in the numerator that will cancel out that M (pick an N that’s divisible by the M).
M = 4
N = 40
Make sure you’re keeping N and M constraints in mind.
3*40/4 = integer
40/4 = integer
No
For the no we’ll do something similar but this time pick an N not divisible by the M. Let’s pick an M that will be at least partially cancelled out by the 3. So 6 would be a good M. And then just pick an even N (to cancel the 2 in 6) not divisible by 6. So N could be 14.
3*14/6 = integer
14/6 is not equal to an integer.
For statement one we have a yes and a no so it’s insufficient.
(2) It is possible to assign each of 13N students to one of M classrooms so that each classroom has the same number of students assigned to it.
M = 4, 5, 6, 7, 8, 9, 10, 11, 12 N > 13
Statement two looks very similar BUT just because statements look similar doesn’t mean they”ll have the same outcome. The details matter. Still, we can use the same procedure setting up a yes/no to test sufficiency.
Yes
So 13N/M = integer
So M could be 7 and N 14.
13*14/7 = integer
No
Given the constraint this is impossible. 13/M will never be an integer because M can only be 4, 5, 6, 7, 8, 9, 10, 11, 12. Eh?
So what!
Well, if M can’t be cancelled out by the 13 then how is it going to disappear from the denominator to leave an integer?
The N has fully cancel the M. Aha! So, given this constraint N/M must be an integer.
Statement two is sufficient.
B.
Fully Worked out Diagram of the Solution for: A school administrator will assign each student…
Video Explanation for: A school administrator will assign each student…
Additional GMAT Data Sufficiency Divisibility Practice Questions
GMAT Data Sufficiency Divisibility Question of the Day 1
GMAT Data Sufficiency Divisibility Question of the Day 2
GMAT Data Sufficiency Divisibility Question of the Day 3