A certain characteristic in a large population has a distribution that is symmetric about the mean m. If 68 percent of the distribution lies within one standard deviation d of the mean, what percent of the distribution is less than m + d? GMAT Explanation, Video Solution, and Additional Practice!
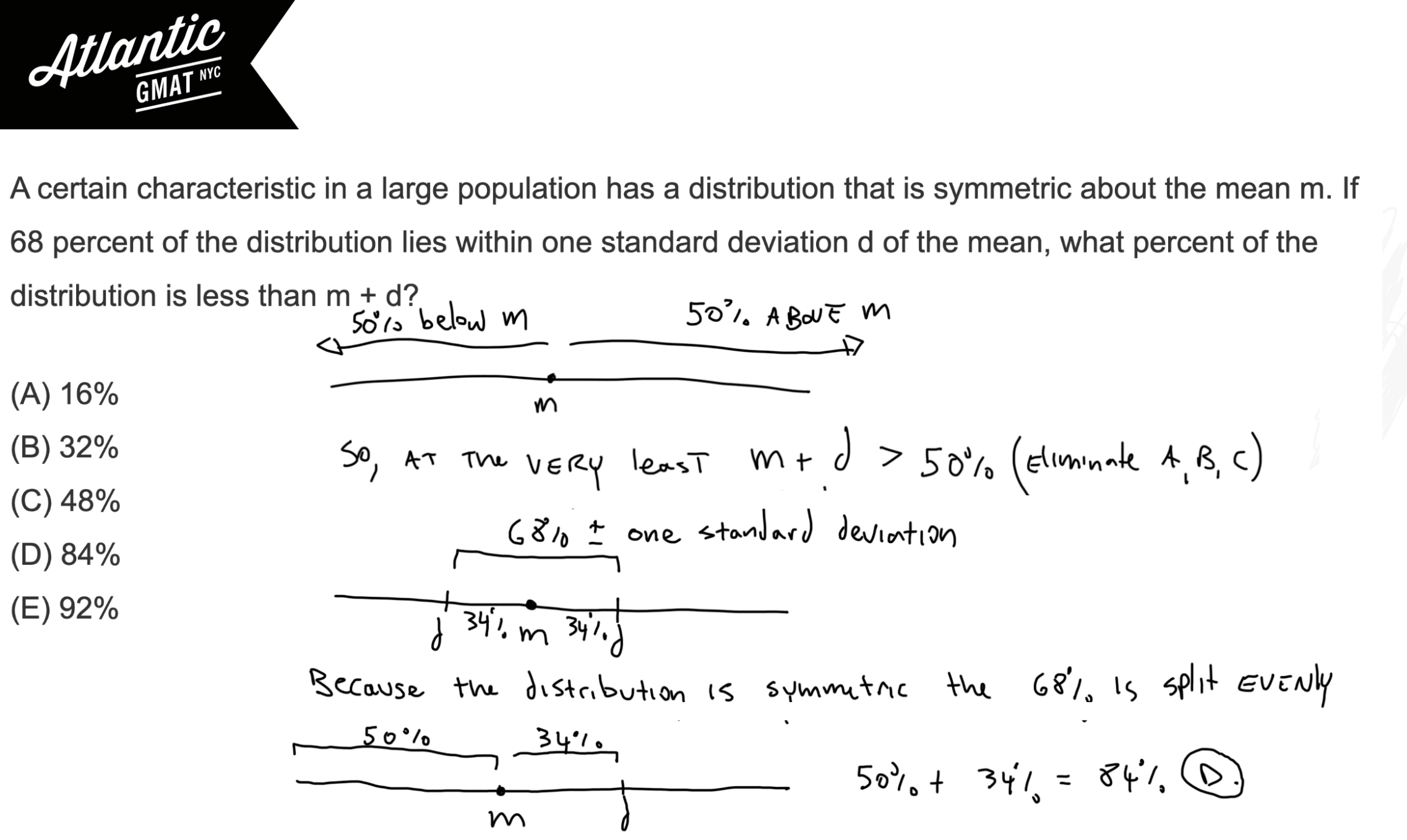
A certain characteristic in a large population has a distribution that is symmetric about the mean m. If 68 percent of the distribution lies within one standard deviation d of the mean, what percent of the distribution is less than m + d?
(A) 16%
(B) 32%
(C) 48%
(D) 84%
(E) 92%
The good news is: you do not need to memorize the standard deviation formula. It is a waste of time and will not help you on the GMAT. Unluckily when I first studied for the exam I was told by my GMAT tutor to memorize that beast. Yeah. Not good. You do though need to have a sense for what standard deviation means and how to work with it in an equation with mean.
Standard deviation is a measure of spread. How spread out is the set. If the set is concentrated around the mean then it has a small spread or small standard deviation. If it is a very diffuse set with numbers scattered way out then the set will have a greater standard deviation. That’s about it in terms of what you need to know conceptually.
Beyond that you need to understand how to work an equation with standard deviation and mean. Let’s say x is within one standard deviation of the mean that indicates that,
x is between: Mean – standard deviation and Mean + Standard deviation.
If x is two standard deviations above the mean that indicates: x = mean + 2*standard deviation.
If x is one standard deviation below the mean then x = mean – standard deviation. You get the point.
Back to the question: A certain characteristic in a large population has a distribution that is symmetric about the mean m. If 68 percent of the distribution lies within one standard deviation d of the mean, what percent of the distribution is less than m + d?
Distribution is symmetric about the mean??? The distribution is split evenly. 50% above the mean. 50% below the mean.
And then 68 percent of the distribution is within one standard deviation. Aha! So that’s what we were talking about above. If within one SD then it’s 1 SD above and 1 SD below meaning the 68 percent is divided in half, 34% above and 34% below.
We want to know: what percent of the distribution is less than m + d? That translates to, what percent is +1 standard deviation or below. So we know that between the mean and +1 standard deviation is 34% of the distribution. And we know that below the mean is 50% of the distribution (symmetric) so 34% + 50% = 84%.
Correct Answer: D
Video Solution: A certain characteristic in a large population has a distribution that is symmetric about the mean m. If 68 percent of the distribution lies within one standard deviation d of the mean, what percent of the distribution is less than m + d?
Additional GMAT Statistics Standard Deviation Practice Questions
Here’s a GMAT statistics standard deviation question from the GMAT Prep Tests. It’s a bit more straightforward but good practice for understanding the mechanics of mean and standard deviation: A vending machine is designed to dispense 8 ounces of coffee into a cup.
And finally a challenging Data Sufficiency GMAT Question of the Day with standard deviation strategies.