If 12 men and 16 women can do a piece of work in 5 days and 13 men and 24 women can do it in 4 days, how long will 7 men and 10 women take to do it? GMAT Explanation + Additional Examples!

If 12 men and 16 women can do a piece of work in 5 days and 13 men and 24 women can do it in 4 days, how long will 7 men and 10 women take to do it?

(A) 4.2 days

(B) 6.8 days

(C) 8.3 days

(D) 9.8 days

(E) 10.2 days

 

Define the question: How long will 7 men and 10 women take to do it?

Looks like a slightly non-standard cooperative rate question.’

 

Organize the information: Let’s make a system of equations

We can use our rate T’s to lay things out but ultimately it’s looking like we’re going to treat this more as a system of equations.

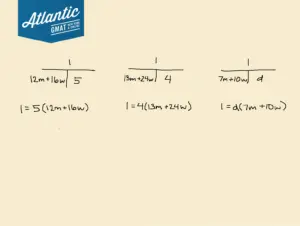
Let’s call the rate that men work at ‘m’, the rate that women work at ‘w’, and the number of days we are looking for ‘d’.

 

From this, we can see the following:

1 = 5 * (12m + 16w)

1 = 4 * (13m + 24w)

1 = d * (7m + 10w)

 

Now we need to work the equations to solve for d.

 

Time for some algebra:

The first two equations are both equal to so let’s just set them equal to each other and simplify.

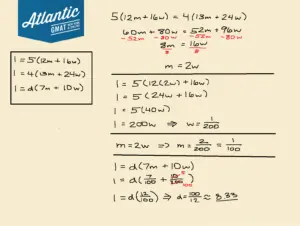
5 * (12m + 16w) = 4 * (13m + 24w)

60m + 80w = 52m + 96w

8m = 16w

m = 2w

 

Now, we’ve solved for m in terms of w. Let’s substitute that back into our first equation to solve for w.

1 = 5 * (12 * (2w) + 16w)

1 = 5 * (24w + 16w)

1 = 5 * 40w

1 = 200w

w = 1/200

 

Great: We have the womens’ rate.

Since m = 2w then the rate for men is 2/200, or 1/100.

We can plug these into the third equation to solve for d:

1 = d * (7m + 10w)

1 = d * (7/100 + 10/200)

1 = d * (7/100 + 5/100)

1 = d * 12/100

d = 100/12 = 8 ⅓

 

How long will 7 men and 10 women take to do it? 8 ⅓ days. 

Correct Answer: C

 

Here is all of that worked out: